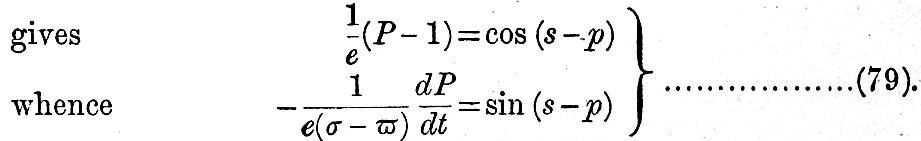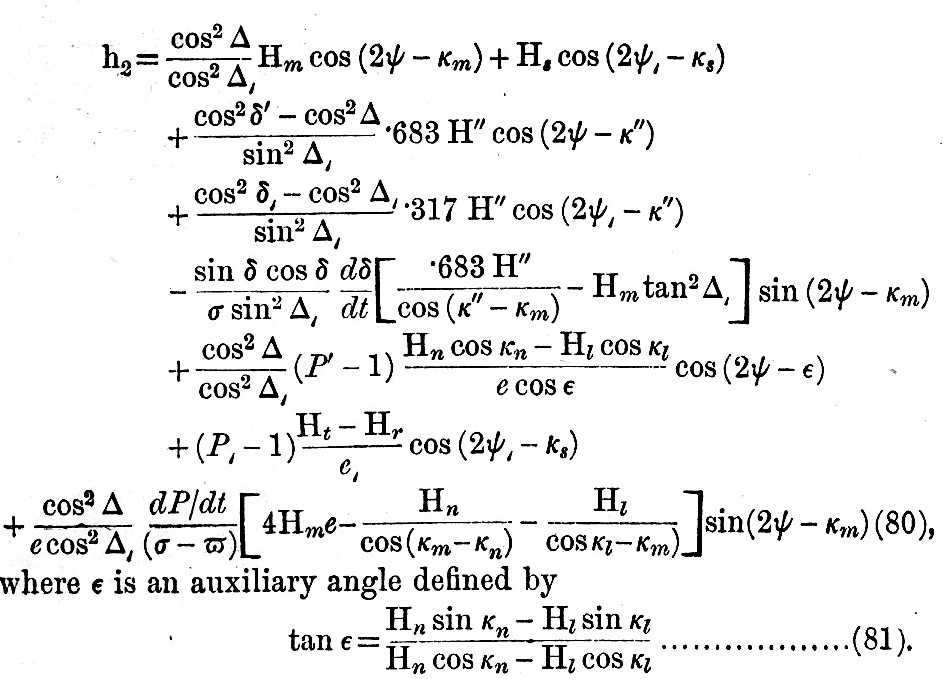V. SYNTHETIC METHOD (cont.)

29. Semi-Diurnal Tides

The process adopted is to replace the mean longitudes and elements of the orbit in each term of the harmonic development of the schedules of § 23 by hour-angles, declinations, and parallaxes.

At the time t (mean solar time of port reduced to angle) let α, δ, ψ R.A., declination, and hour-angle, and l longitude measured from the "intersection." These and other symbols when written with subscript accent are to apply to the sun. Then ν being the R.A. of the intersection, we have from the right-angled spherical triangle of which the sides are l, δ, α - ν the relations

Now s - ξ is the mean longitude measured from the intersection and s - p is the mean anomaly; hence approximately

From (74) and (75) we have approximately

Now, h being the mean longitude, t + h is the sidereal hour-angle, and

ψ = t + j - a

Hence

Again, if we put

we have approximately from (74) and (75)

Obviously Δ is such a declination that sin² Δ is the mean value of sin² δ during a lunar month. Again, if P be the ratio of the parallax to her mean parallax, the equation to the ellipse described

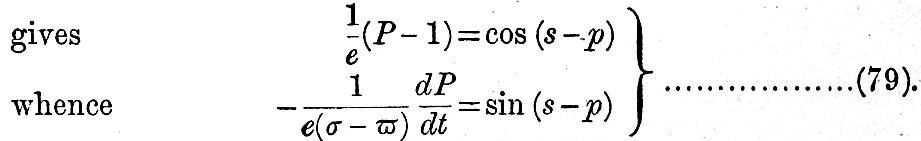
Now it appears in schedule A of § 23 that the arguments of all the lunar semi-diurnal tides are of the form 2(t + h - ν)±2(s - ξ) or ±(s - p). It is clear, therefore, that the cosines of such angles may by the relations (76), (78), (79) be expressed in terms of hour-angles, declinations, and parallaxes. Also by means of (77) we may introduce Δ in place of I in the coefficients of each term. An approximate formula for Δ is 16°.51 + 3°.44 cos N - 0°.19 cos 2N. In the Report to the British Association for 1885, the details of the processes indicated are given.

Before giving the formula it must be remarked that the result is expressed more succinctly by the introduction of the symbol δ″ to denote the declination at a time earlier than that of observation by an interval which may be called the "age of the declinational inequality," and is computed from the formula tan (κ″ - κ_m /2σ or 52^h.2 tan (κ″ - κ_m). Similarly, it is convenient to introduce P′ to denote the value of P at a time earlier than that of observation by the "age of the parallactic inequality," to be computed from or 105^h.3 tan (κ_m - κ_n). These two "ages" probably do not differ in general much from a third period, computed from (κ₈ - κ_m)/2/(σ - η), which is called the "age of the tide."

The similar series of transformations when applied to the solar tides to simpler results, because is a constant, being 16°·33, and the "ages" may be treated as zero; besides the terms depending on and are negligible. If now we denote by h₂ the height of water with reference to mean watermark, in so far as the height is affected by the harmonic tides M₂, S₂, K₂, N, L, T, R, [Footnote 367-1] the harmonic expression is transformed into

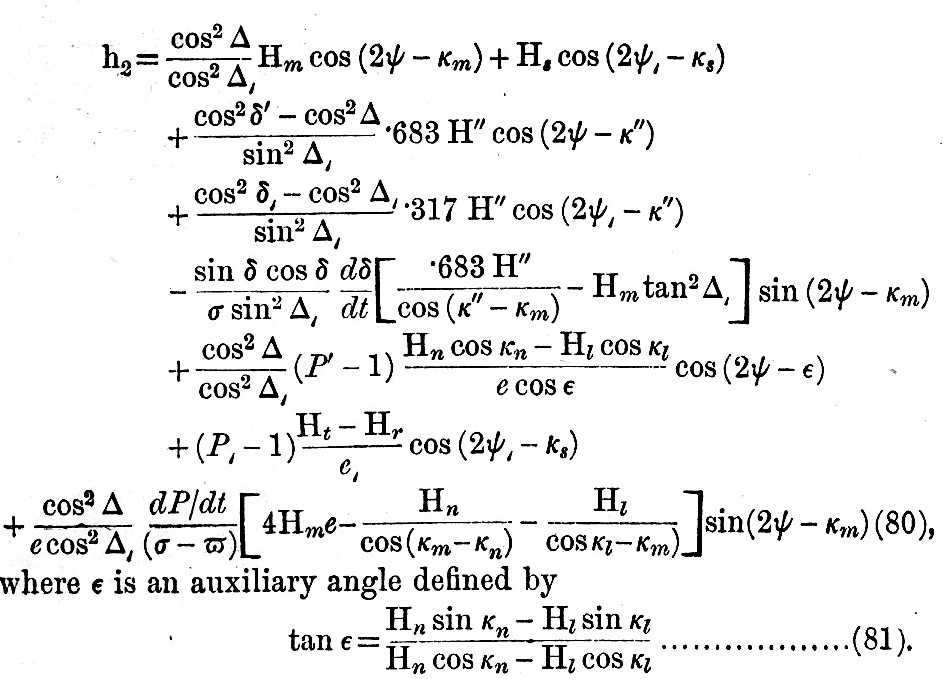
The first two terms are the principal tides, and the physical origin of the remaining small terms is indicated by their involving 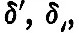The terms in dδ/dt and dP/dt are generally smaller than the others.

The approximation may easily be carried further. But the above is in some respects a closer approximation than the expression from which it is derived, since the hour-angles, declinations, and parallaxes necessarily involve all the lunar and solar inequalities.


Footnote

367-1 R is the smaller solar elliptic tide bearing the same relation to T that L does to N amongst the lunar tides. It was omitted as unimportant in schedule [B.i.] of § 23.






Read the rest of this article:
Tides - Table of Contents